Hey everyone, I’ve been working on something pretty interesting lately and I thought I’d share my experience with you all. It’s about setting up a baseball field, and more specifically, about the shape of the baseball diamond. I had always known it was called a “diamond,” but I never really gave much thought to its exact shape until now.
So, I started this little project because our local community was planning to set up a new baseball field. We didn’t have much space to work with, so every foot counted. That’s when I decided to dig a bit deeper into the dimensions of a baseball diamond. I thought, “It’s just a square, how hard can it be?” Boy, was I in for a surprise!
Getting Started
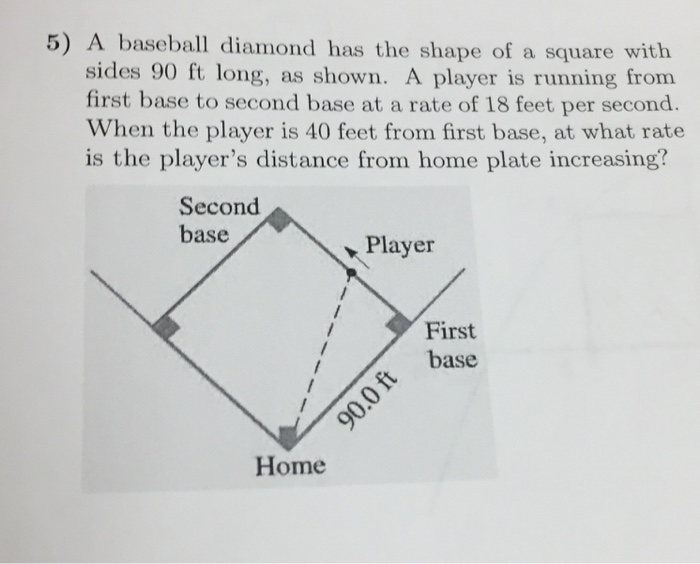
First off, I learned that a baseball diamond is, in fact, a perfect square. Each side of this square is supposed to be exactly 90 feet long. I went online to find some more info about this, and the internet gave me tons of pages that said the same thing, “The diamond is a square that measures 90ft on all sides.” Alright, I got it, it’s 90 feet.
In our case, due to space limitations, we could only afford 80 feet per side. “It’s close enough,” I thought, and we started marking the ground. We measured out the first side, 80 feet, no problem. Then the second side, another 80 feet. We were feeling pretty good about ourselves at this point.
The Realization
But then came the tricky part. When we started to measure from the home plate to the second base, that’s where we hit a snag. It wasn’t just a simple side measurement anymore; it was the diagonal of the square. And as anyone who’s messed around with squares knows, the diagonal is longer than the sides. One source I found online even mentioned something about the Pythagorean theorem, “a baseball diamond has the shape of a square, a line from third base to first base would be the diagonal of this square. The sides (a and b) of the square are 90 feet in length so we can use the Pythagorean theorem to find the length of the diagonal (c).” What was that? I don’t remember anything called “Pythagorean theorem”…
- I had to brush up on some old-school math.
- Turns out, to find the diagonal of a square, you can’t just use the side length.
- There’s this whole formula involved, something about the square root of the sum of the squares of the sides.
It took me a while, and some help from a friend who’s a bit of a math whiz, to figure out that the diagonal we needed was not just 80 feet, but approximately 113 feet for a standard 90-foot diamond, and around 113 feet for our 80-foot one. We had to adjust our layout quite a bit. It wasn’t as straightforward as I had initially thought.
Wrapping Up
In the end, we managed to set up our baseball diamond, a bit smaller than the standard, but hey, it’s a perfect square! It was a great learning experience. I realized that even something as seemingly simple as setting up a baseball diamond has its own complexities. It’s not just about knowing the side lengths; it’s about understanding the geometry of the shape you’re working with.
I felt a real sense of accomplishment once we were done. It wasn’t just about the physical work; it was about the problem-solving and learning that went into it. Plus, now we have a cool little baseball field where the kids can play, and I can proudly say I played a part in making it happen.
So, that’s my story. I hope you guys found it somewhat interesting or at least a bit educational. It’s funny how much you can learn from just setting up a baseball diamond. I guess it’s true what they say, “You learn something new every day!”






















